Polyiamonds attaining extremal topological properties, part I

Abstract

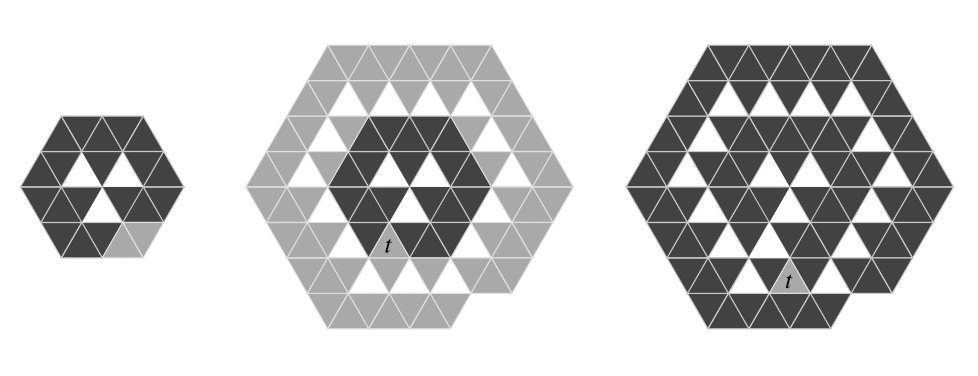
We consider two optimization questions with respect to polyiamonds. What is the maximum number of holes that a polyiamond with $n$ tiles can enclose, and what is the minimum number of tiles required to construct a polyiamond with $h$ holes? These numbers will be given by the sequences $f_{\triangle}(h)$ and $g_{\triangle}(h)$, respectively. In this paper, we construct a sequence of polyiamonds with $h_k = \frac{3}{2}(k^2-k)$ holes and $n_k=\frac{1}{2}(9k^2+3k-4)$ tiles such that $g_{\triangle}(n_k)=h_k$. Furthermore, these polyiamonds all attain a specific set of efficient geometric and topological properties.